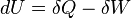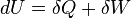Assumptions in the Kenitic Theory of Gases

The kinetic theory of gases is the study of the microscopic behavior of molecules and the interactions which lead to macroscopic relationships like the ideal gas law.

The study of the molecules of a gas is a good example of a physical situation where statistical methods give precise and dependable results for macroscopic manifestations of microscopic phenomena. For example, the pressure, volume and temperature calculations from the ideal gas law are very precise. The average energy associated with the molecular motion has its foundation in the Boltzmann distribution, a statistical distribution function. Yet the temperature and energy of a gas can be measured precisely.

Kinetic Theory of Gases

Temperature and pressure are macroscopic properties of gases. These properties are related to molecular motion, which is a microscopic phenomenon. The kinetic theory of gases correlates between macroscopic properties and microscopic phenomena. Kinetics means the study of motion, and in this case motions of gas molecules.

At the same temperature and volume, the same numbers of moles of all gases exert the same pressure on the walls of their containers. This is known as Avogadros principle. His theory implies that same numbers of moles of gas have the same number of molecules.

Common sense tells us that the pressure is proportional to the average kinetic energy of all the gas molecules. Avogadros principle also implies that the kinetic energies of various gases are the same at the same temperature. The molecular masses are different from gas to gas, and if all gases have the same average kinetic energy, the average speed of a gas is unique.

Based on the above assumption or theory, Boltzmann (1844-1906) and Maxwell (1831-1879) extended the theory to imply that the average kinetic energy of a gas depends on its temperature.

They let u be the average or root-mean-square speed of a gas whose molar mass is M. Since N is the Avogadro's number, the average kinetic energy is (1/2) (M/N) u² or

          M        3R T     3
K.E.  = --- u2   = ---- = --- k T
         2 N        2 N     2

Note that M / N is the mass of a single molecule. Thus,

u = (3k N T / M)^1/2
= (3 R T / M)^1/2.

where k (= R/N) is the Boltzmann constant. Note that u so evaluated is based on the average energy of gas molecules being the same, and it is called the root-mean-square speed; u is not the average speed of gas molecules.

Calculate the kinetic energy of 1 mole of nitrogen molecules at 300 K?

Solution

Assume nitrogen behave as an ideal gas, then E_k = ³/₂ R T
= (³/₂) 8.3145 J/(mol K) * 300 K
= 3742 J / mol (or 3.74 kJ/mol)

Equivalence of Kelvin-Planck and Clausius Statements

The Clausius statement implies the Kelvin-Planck statement: -

Suppose that we violated the Kelvin-Planck statement and constructed a magic heat engine. Then we could take its work output and use it to power a (non-magical) fridge, which draws heat from a cooler thermal reservoir and discharges this heat into the thermal reservoir that the magic heat engine runs off, as shown in the diagram to the right. Then this combined system of the fridge and the magic heat engine is a magic fridge, since it moves heat from a lower temperature to a higher temperature while having no other effect on its environment - in particular, without having an external power source.

So if we had a magic heat engine, we could have a magic fridge. Therefore, the statement that you can't have a magic fridge implies that you can't have a magic heat engine.

The Kelvin-Planck statement implies the Clausius inequality: -

We now wish to prove that the statement that you can't have a magic heat engine implies the Clausius inequality - that for any closed, stationary system operating in a cycle

where Q is as usual the heat transferred to the system and T is the temperature at the boundary of the system.

We shall prove this with the assistance of the piece of imaginary equipment shown in the diagram below. We choose any closed, stationary system (hereafter referred to as "the chosen system"). We imagine that the chosen system is attached to a reversible system which is connected to a thermal reservoir, as shown on the diagram.

By convention, the diagram shows heat flowing in to the chosen system and work coming out of it. We emphasize, however, that this is not a restriction on what the chosen system is allowed to do: it merely means that work input or heat output will have negative sign.

It is important to note that the inequality we're going to derive relates only to the boundary temperature and heat flow of the chosen system, and is therefore true whether or not the chosen system is connected to a reversible system which is connected to a thermal reservoir. The reversible system and the thermal reservoir are, so to speak, intellectual scaffolding, which will be discarded after we have produced our result.

So, consider the diagram. The combined system of the chosen system and the reversible system to which it's attached is, like everything else, subject to the First Law of Thermodynamics, which we give for the combined system in its differential form: δQ_r - δW = dE.

Now, as the reversible system is reversible, then if we are using a Thermodynamic Temperature Scale (eg degrees kelvin) then by the definition of a thermodynamic temperature scale we have δQ_r / δQ = T_r / T.

We can rearrange this as δQ_r = T_r δQ / T and substitute this into the First Law equation above, giving us: T_r δQ / T - δW = dE

For convenience, we shall assume that the reversible system performs an whole number of cycles in the same time that the chosen system takes to execute one. Now, let's integrate the equation above over one cycle of the chosen system.

Of course, is simply W. To evaluate , recall that over a cycle, the combined system can neither gain nor lose energy - or it wouldn't be a cycle - and so . This gives us

which we shall rearrange to give

Now, here's the clever part. There is no such thing as a magic heat engine. That's the Kelvin-Planck statement of the Second Law, and the whole thing we're basing our argument on.

Now, look at the diagram given above, and observe that the combined system is connected to only one thermal reservoir. If the net work output W was greater than zero, the combined system would be a magic heat engine. Therefore, the net work output W of the combined system is less than or equal to zero, and so

Finally, we note that T_r is a constant (being the temperature of a thermal reservoir) and positive, since we are using a thermodynamic temperature scale. So we can divide both sides of the inequality through by T_r, giving us

Second Law of Thermodynamics Statement by Clausius

The statement of second law of thermodynamics made by Clausius goes like this, “It is impossible to construct a device which, operating in a cycle, will produce no effect other than the transfer of heat from a colder to a hotter body.” The statement says that to transfer the heat from low temperature to high temperature reservoir some external work should be done on the cycle. This statement has been the basis for the working for all refrigerators, heat pumps and air-conditioners.

Second Law of Thermodynamics Statement by Kelvin-Planck

Kelvin-Planck’s statement is based on the fact that the efficiency of the heat engine cycle is never 100%. This means that in the heat engine cycle some heat is always rejected to the low temperature reservoir. The heat engine cycle always operates between two heat reservoirs and produces work.

The statement made by Kelvin-Planck for third law of thermodynamics says, “It is impossible for a heat engine to produce net work in a complete cycle if it exchanges heat only with bodies at a single fixed temperature.” Thus to produce the work the cycle should exchange heat with two reservoirs which are a different temperatures. The high temperature reservoir is called as source and low temperature reservoir is called as sink.

W = Q_H – Q_C

As per the above statement the net work will be produced in the cycle as long as there is difference in temperature between the source and sink. In due course of time if source loses too much heat and sink gains too much heat and their temperatures become equal, the net work produced in the cycle will be zero.

The Kelvin-Planck statement tells the condition for producing work within the cycle, the Carnot’s statement tells maximum work or efficiency that can be obtained within the cycle.

The ratio of the maximum mechanical work obtain to the total heat supplied to the engine is known as maximum thermal efficiency (η_max) of the engine. Mathematically

η_max = Maximum work obtain / Total heat supplied

= Q_H – Q_C/Q_H = 1 – Q_C/Q_H = 1 – T_C/T_H

For a reversible engine, Q_H/T_H = Q_C/T_C

Limitations of first law of thermodynamics

1. When a closed system undergoes a thermodynamic cycle, the net heat transfer is equal to the net work transfer. This statement does not specify the direction of flow of heat and work (i.e. whether the heat flows from a hot body to a cold body or from a cold body to a hot body). It also does not give any condition under which these transfers take place.
2. The heat energy and mechanical work are mutually convertible. Though the mechanical work can be fully converted into heat energy, but only a part of heat energy can be converted into mechanical work. This means that the heat energy and mechanical work are not fully mutually convertible. In other words, this is a limitation on the conversion of one form of energy into another form.

Heat and Work - A Path Function

Path function and Point function are introduced to identify the variables of thermodynamics.

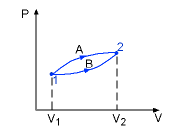
• Path function: Their magnitudes depend on the path followed during a process as well as the end states. Work (W), heat (Q) are path functions.
  Process A: W_A = 10 kJ
  Process b: W_B = 7 kJ
  
  
• Point Function: They depend on the state only, and not on how a system reaches that state. All properties are point functions.
  Process A: V₂ - V₁ = 3 m³
  Process B: V₂ - V₁ = 3 m³

Heat:-

Heat is energy transferred from one system to another solely by reason of a temperature difference between the systems. Heat exists only as it crosses the boundary of a system and the direction of heat transfer is from higher temperature to lower temperature.

For thermodynamics sign convention, heat transferred to a system is positive; Heat transferred from a system is negative.

The heat needed to raise a object's temperature from T₁ to T₂ is:

Q = c_p m (T₂ - T₁)

where
c_p = specific heat of the object (will be introduced
in the following section)
m = mass of the object

Unit of heat is the amount of heat required to cause a unit rise in temperature of a unit mass of water at atmospheric pressure.

• Btu: Raise the temperature of 1 lb of water 1 ^oF
• Cal: Raise the temperature of 1 gram of water 1 ^oC

J is the unit for heat in the S.I. unit system. The relation between Cal and J is

1 Cal = 4.184 J

Notation used in this book for heat transfer:

• Q : total heat transfer
• : the rate of heat transfer (the amount of heat transferred per unit time)
• δQ: the differential amounts of heat
• q: heat transfer per unit mass

Modes of Heat Transfer: -

Conduction: Heat transferred between two bodies in direct contact.

Fourier's law:

If a bar of length L was put between a hot object T_H and a cold object T_L , the heat transfer rate is:

where
k_t = Thermal conductivity of the bar
A = The area normal to the direction of heat
transfer

Convection: Heat transfer between a solid surface and an adjacent gas or liquid. It is the combination of conduction and flow motion. Heat transferred from a solid surface to a liquid adjacent is conduction. And then heat is brought away by the flow motion.

Newton's law of cooling:

where
h = Convection heat transfer coefficient
T_s = Temperature of the solid surface
T_f = Temperature of the fluid

The atmospheric air motion is a case of convection. In winter, heat conducted from deep ground to the surface by conduction. The motion of air brings the heat from the ground surface to the high air.

Radiation: The energy emitted by matter in the form of electromagnetic waves as a result of the changes in the electronic configurations of the atoms or molecules.

Stefan - Boltzmann law:

where
σ = Stefan - Boltzmann constant
ε = emissivity
T_s = Surface temperature of the object

Solar energy applications mainly use radiation energy from the Sun.

The three modes of heat transfer always exist simultaneously. For example, the heat transfer associated with double pane windows are:

• Conduction: Hotter (cooler) air outside each pane causes conduction through solid glass.
• Convection: Air between the panes carries heat from hotter pane to cooler pane.
• Radiation: Sunlight radiation passes through glass to be absorbed on other side.

Please view heat transfer books for details of modes of heat transfer.

Work: -

Work is the energy transfer associated with a force acting through a distance.

Dot product means the distance along the force's direction. For example, if a car runs at a flat road, its weight does zero work because the weight and the moving distance have a 90^o angle.

Like heat, Work is an energy interaction between a system and its surroundings and associated with a process.

In thermodynamics sign convection, work transferred out of a system is positive with respect to that system. Work transferred in is negative.

Units of work is the same as the units of heat.

Notation:

• W : total work
• δW: differential amount of work
• w: work per unit mass
• : Power, the work per unit time

Expansion and Compression Work: -

A system without electrical, magnetic, gravitational motion and surface tension effects is called a simple compressible system. Only two properties are needed to determine a state of a simple compressible system.

Considering the gas enclosed in a piston-cylinder device with a cross-sectional area of the piston A.

Initial State:

• Pressure P₁
• Volume V₁
  

Finial State:

• Pressure P₂
• Volume V₂

Then a work between initial and final states is: Pressure P, Volume V. Let the piston moving ds in a quasi-equilibrium manner. The differential work done during this process is:

δW = F ds = P A ds = P dV

The total work done during the whole process (from state (P₁,V₁) to state (P₂,V₂)) is:

This quasi-equilibrium expansion process can be shown on a P-V diagram. The differential area dA is equal to P dV. So the area under the process curve on a P-V diagram is equal, in magnitude, to the work done during a quasi-equilibrium expansion or compression process of a closed system.

Heat and Work - A Path Function: -

There are many similarities between heat and work. These are

1. The heat and work are both transient phenomena. The systems do not possess heat or work. When a system undergoes aa change, heat transfer or work done may occur.
2. The heat and work are boundary phenomena. They are observed at the boundary of the system
3. The heat and work represent the energy crossing the boundary of the system.
4. The heat and work are path functions and hence they are inexact differentials. They are written as δQ and δW.

Three Laws of Thermodynamics

There are three laws of thermodynamics such as:

1. Zeroth Law of Thermodynamics
2. First Law of Thermodynamics and
3. Second Law of Thermodynamics

This Laws are discussed in detail, as follows

Zeroth Law of Thermodynamics: -

When two systems are each in thermal equilibrium with a third system, then two system are also in thermal equilibrium with each other.

A system is said to be in thermal equilibrium when its temperature does not change over time. Let A, B, and C be distinct thermodynamic systems or bodies. The zeroth law of thermodynamics can then be expressed as:

"If A and B are each in thermal equilibrium with C, A is also in thermal equilibrium with B."

The preceding sentence asserts that thermal equilibrium is a Euclidean relation between thermodynamic systems. If we also grant that all thermodynamic systems are (trivially) in thermal equilibrium with themselves, then thermal equilibrium is also a reflexive relation. Relations that are both reflexive and Euclidean are equivalence relations. One consequence of this reasoning is that thermal equilibrium is a transitive relation between the temperature T of A, B, and C:

For example, if two systems of ideal gas are in equilibrium, then P₁V₁/N₁ = P₂V₂/N₂ where P_i is the pressure in the ith system, V_i is the volume, and N_i is the "amount" (in moles, or simply the number of atoms) of gas.

First Law of Thermodynamics: -

This law may be stated as follows:
a) "The heat and mechanical work are mutually convertible". According to this law, when a closed system undergoes a thermodynamic cycle, the net heat transfer is equal to the net work transfer.

b) "The First Law states that energy cannot be created or destroyed". The amount of energy lost in a steady state process cannot be greater than the amount of energy gained. This is the statement of conservation of energy for a thermodynamic system.

All laws of thermodynamics but the First are statistical and simply describe the tendencies of macroscopic systems. For microscopic systems with few particles, the variations in the parameters become larger than the parameters themselves, and the assumptions of thermodynamics become meaningless. The First Law, i.e. the law of conservation, has become the most secure of all basic laws of science.

The first law of thermodynamics basically states that a thermodynamic system can store or hold energy and that this internal energy is conserved. Heat is a process by which energy is added to a system from a high-temperature source, or lost to a low-temperature sink. In addition, energy may be lost by the system when it does mechanical work on its surroundings, or conversely, it may gain energy as a result of work done on it by its surroundings. The first law states that this energy is conserved: The change in the internal energy is equal to the amount added by heating minus the amount lost by doing work on the environment. The first law can be stated mathematically as:

where dU is a small increase in the internal energy of the system, δQ is a small amount of heat added to the system, and δW is a small amount of work done by the system.

The only difference here is that δW is the work done on the system. So, when the system (e.g. gas) expands the work done on the system is − PdV whereas in the previous formulation of the first law, the work done by the gas while expanding is PdV. In any case, both give the same result when written explicitly as:

And also the first law can be expressed as the Fundamental Thermodynamic Relation:

The law of conservation of energy states that the total amount of energy in an isolated system remains constant. A consequence of this law is that energy cannot be created or destroyed. The only thing that can happen with energy in an isolated system is that it can change form, that is to say for instance kinetic energy can become thermal energy. Because energy is associated with mass in the Einstein's theory of relativity, the conservation of energy also implies the conservation of mass in isolated systems (that is, the mass of a system cannot change, so long as energy is not permitted to enter or leave the system).

Second Law of Thermodynamics: -

The entropy of an isolated system not in equilibrium will tend to increase over time, approaching a maximum value at equilibrium.

In a simple manner, the second law states that "energy systems have a tendency to increase their entropy" rather than decrease it. This can also be stated as "heat can spontaneously flow from a higher-temperature region to a lower-temperature region, but not the other way around." (Heat can flow from cold to hot, but not spontaneously—for example, a refrigerator requires electricity.)

A way of looking at the second law for non-scientists is to look at entropy as a measure of disorder. So, for example, a broken cup has less order than an intact one. Likewise, solid crystals, the most organized form of matter, have very low entropy values; and gases, which are highly disorganized, have high entropy values.

The entropy of a thermally isolated macroscopic system never decreases. However, a microscopic system may exhibit fluctuations of entropy opposite to that dictated by the Second Law.